If you use OSMnx in your work, please cite the journal article.
If you use OSMnx in your work, please cite the journal article.
OSMnx is a Python package to retrieve, model, analyze, and visualize street networks from OpenStreetMap. Users can download and model walkable, drivable, or bikeable urban networks with a single line of Python code, and then easily analyze and visualize them. You can just as easily download and work with amenities/points of interest, building footprints, elevation data, street bearings/orientations, and network routing. If you use OSMnx in your work, please download/cite the paper here.
In a single line of code, OSMnx lets you download, model, and visualize the street network for, say, Modena Italy:
import osmnx as ox
ox.plot_graph(ox.graph_from_place('Modena, Italy'))
 Check out the
Check out the  Rentals make up a significant portion of the U.S. housing market, but much of this market activity is poorly understood due to its informal characteristics and historically minimal data trail. The UC Berkeley Urban Analytics Lab collected, validated, and analyzed 11 million Craigslist rental listings to discover fine-grained patterns across metropolitan housing markets in the United States. I’ll summarize our findings below and explain the methodology at the bottom.
Rentals make up a significant portion of the U.S. housing market, but much of this market activity is poorly understood due to its informal characteristics and historically minimal data trail. The UC Berkeley Urban Analytics Lab collected, validated, and analyzed 11 million Craigslist rental listings to discover fine-grained patterns across metropolitan housing markets in the United States. I’ll summarize our findings below and explain the methodology at the bottom.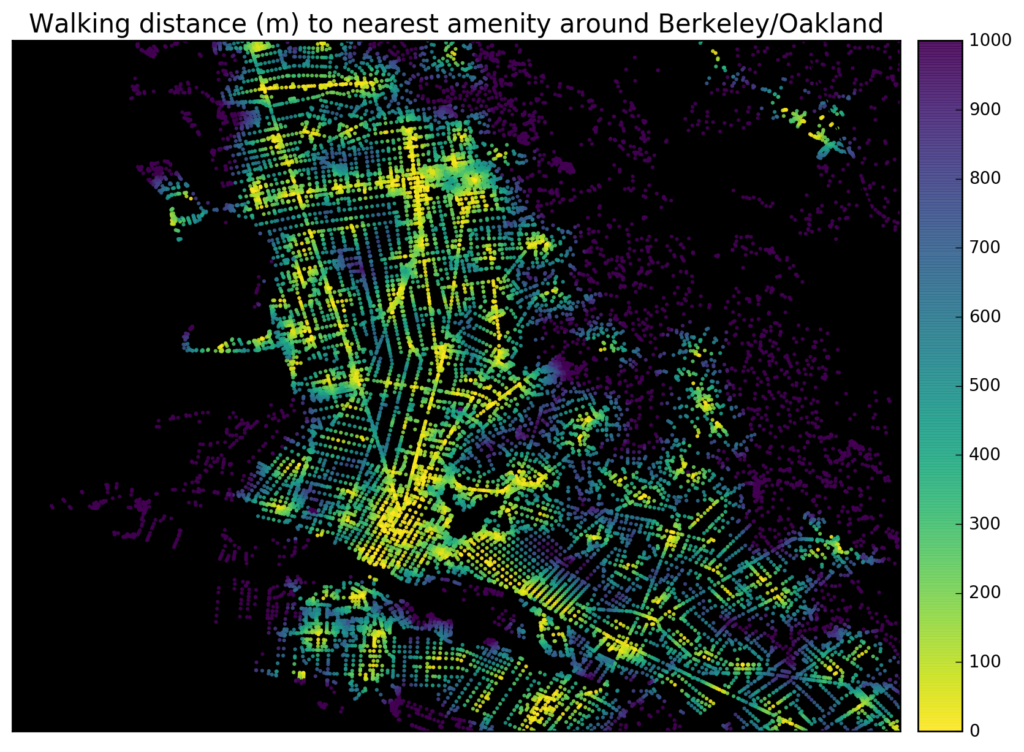
 interactive web maps. To do this, we use the Python programming language, open source analysis and visualization tools, and public data.
interactive web maps. To do this, we use the Python programming language, open source analysis and visualization tools, and public data.
 Using Python to visualize chaos, fractals, and self-similarity to better understand the limits of knowledge and prediction.
Using Python to visualize chaos, fractals, and self-similarity to better understand the limits of knowledge and prediction.